模块和表示论代写 MATH 5735代写 数学作业代写 数学代写

MATH 5735 – Modules and Representation Theory

Assignment 1

模块和表示论代写 1. (9 marks) Recall that an integral domain is a commutative ring (with unity) that has no zero divisors. (a) Prove that if R is an integral domain

(Due Friday, 18 March, 2022, 8pm)

1. (9 marks) 模块和表示论代写

Recall that an integral domain is a commutative ring (with unity) that has no zero divisors.

(a) Prove that if R is an integral domain, then the set of torsion elements in an R-module M (denoted Tor(M)) is a submodule of M.

(b) Give an example of a ring R and an R-module M such that Tor(M) is not a submodule.

(c) Show that if R has zero divisors, then every non-zero R-module has non-zero torsion elements.

2. (9 marks) 模块和表示论代写

Let R be a commutative ring and M an R-module.

(a) Show that Hom_R(R, M) can be given the structure of an R-module in a natural way. (Define this R-module structure explicitly, check that the structure you wrote down is well-defined, then check that it satisfies the axioms of an R-module.)

(b) Show that Hom_R(R, M) and M are isomorphic as R-modules.

(c) Show that End_R(R) and R are isomorphic as rings.

4. (5 marks) 模块和表示论代写

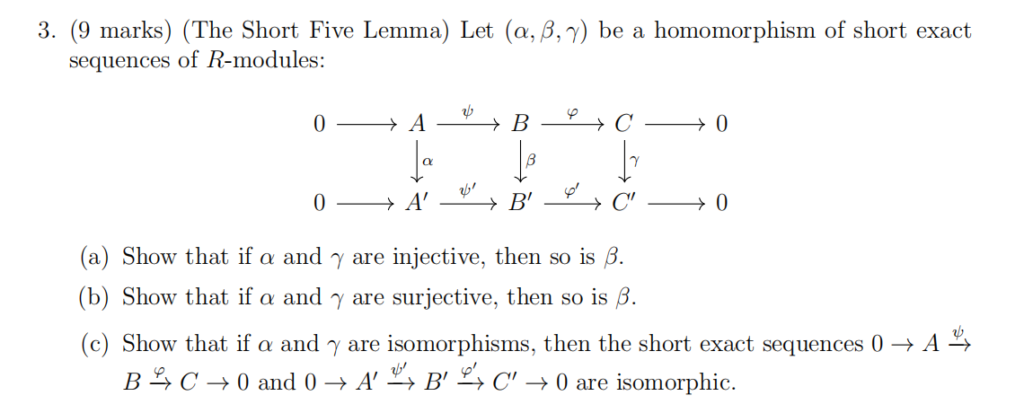
Prove that for every ring R, the following are equivalent.

(a) Every R-module is projective.

(b) Every R-module is injective.

5. (8 marks) 模块和表示论代写

Consider the C[x]-module M := C[x]/(x³ − x² ), where (x³ − x² ) is the ideal in C[x] generated by x³ − x² .

(a) Show that M is finite length by constructing a composition series.

(b) Write down the composition factors of M.

(c) Is M Noetherian? Justify your answer.

(d) Is M Artinian? Justify your answer.

更多代写：java code辅导  gre?proctoru代考  英国物理Final exam代考  term paper怎麼寫  conclusion例子 文章网代写

合作平台：essay代写 论文代写 写手招聘 英国留学生代写